Why Use Manipulatives?
Manipulatives allow students to interpret, comprehend, and represent a wide variety of math concepts.
Explore multi-dimensional teaching and assessment strategies using hands-on materials in our Summer Math Workshop!
Levels of Abstraction
Each manipulative has different levels of difficulty and understanding, which we refer to as a “level of abstraction”. This phrase refers to the level of abstract thinking that is required by a student to successfully use the particular manipulative. For instance, can they understand and explain why a particular block holds a representational value (one blue block has a value of 9) even though it is only one block with no marks on it?
When adding manipulatives to your math curricula, allow students to explore the manipulatives before being asked to solve problems with them. We find daily transition times are great opportunities for students to explore.
The list below provides a progression for the levels of abstraction with the most commonly used manipulatives. These manipulatives are excellent for even the youngest mathematicians! Just keep in mind your assessments should note the difficulty or “level of abstraction” that each manipulative requires of a student.
Wondering how to use manipulatives in your classroom?
Note: We do not sell manipulatives, nor do we have any relationships with manufacturers or vendors. This list is meant solely as a resource.
Common Manipulatives
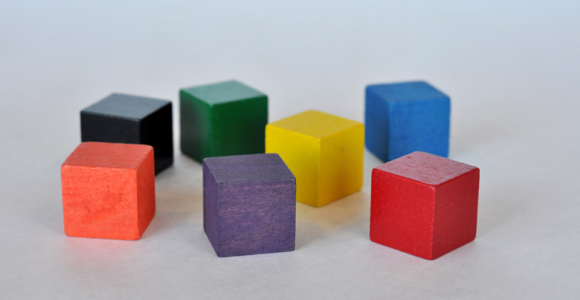
Wooden Cubes
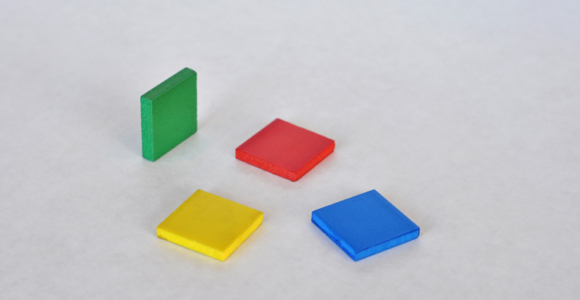
Color Tiles
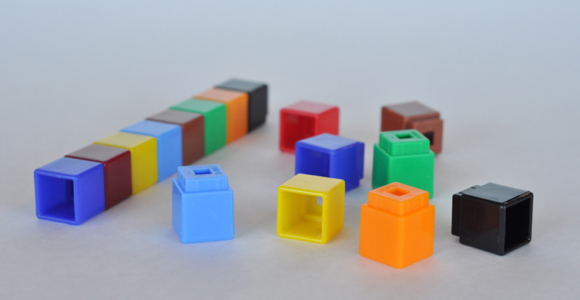
Unifix Cubes
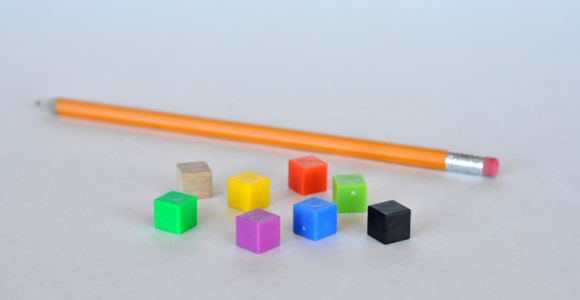
Centimeter Cubes

Multilinks
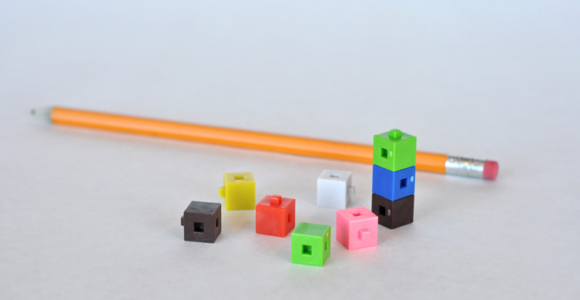
Centimeter Cubes with Connectors
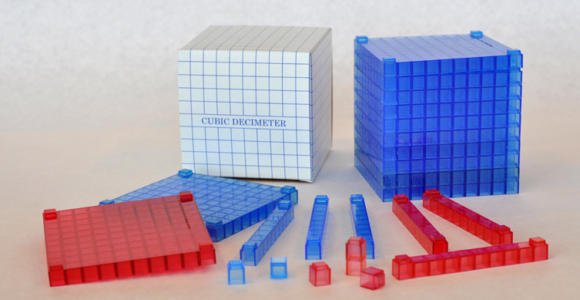
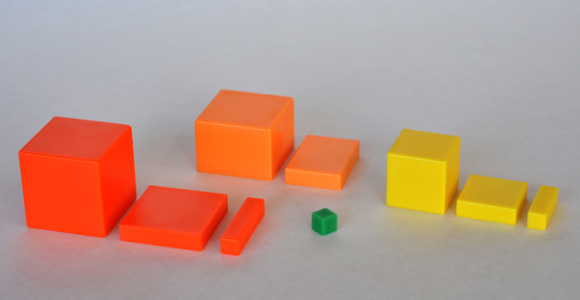
Base Ten Blocks
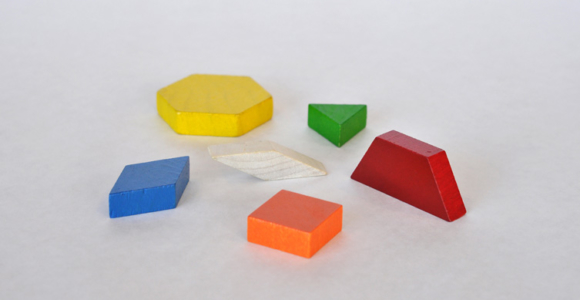
Pattern Blocks

Pentablocks
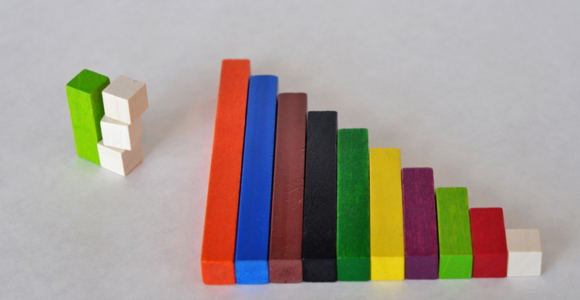
Cuisenaire Rods

Geoblocks
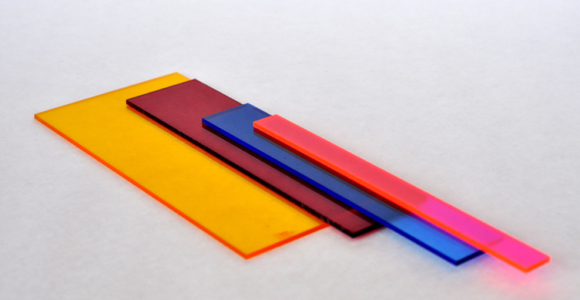
Fraction Tiles
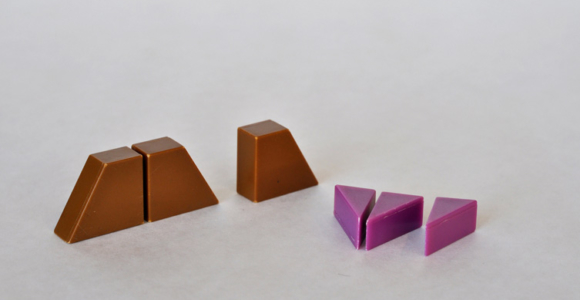
Fractional Pattern Blocks
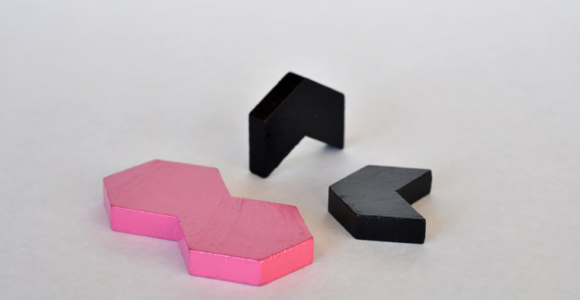
Fractional Pattern Blocks
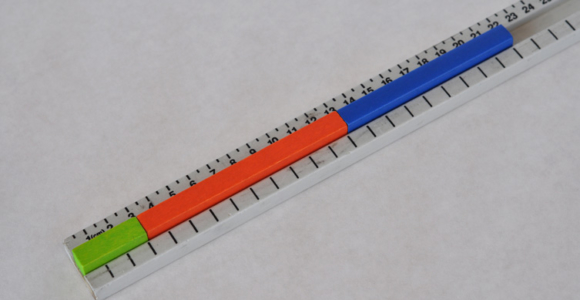
Cuisenaire Rod Counting Track
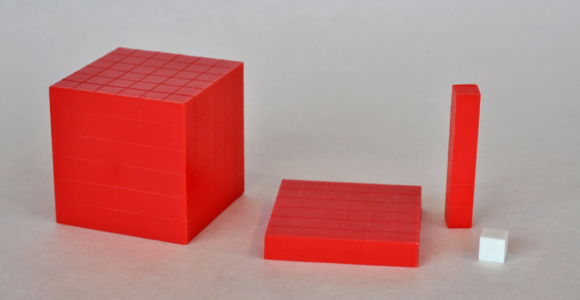
Base Six Blocks
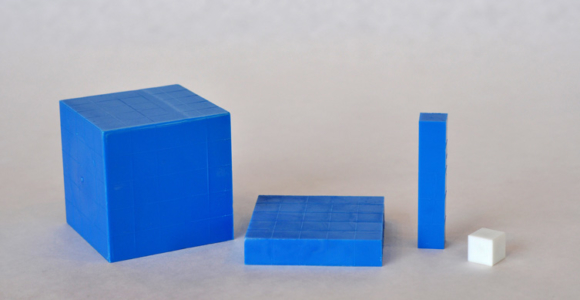
Base Five Blocks
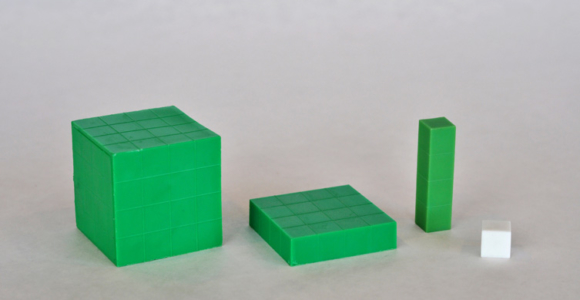
Base Four Blocks
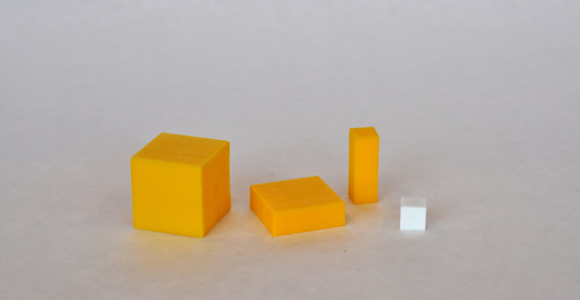
Base Three Blocks
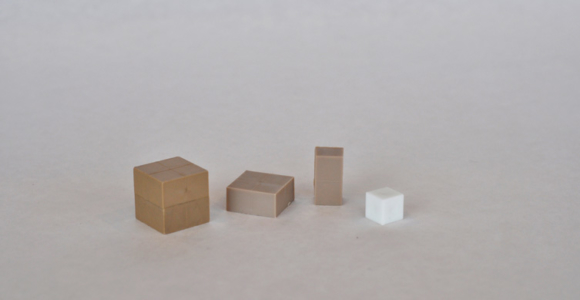
Base Two Blocks
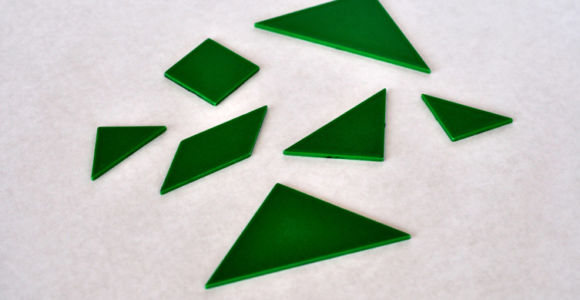
Tangrams
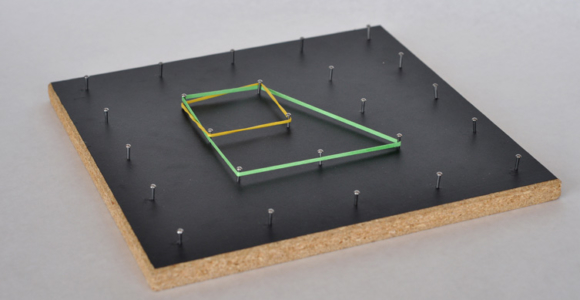
Geoboards

Dice

Stamps & Stencils
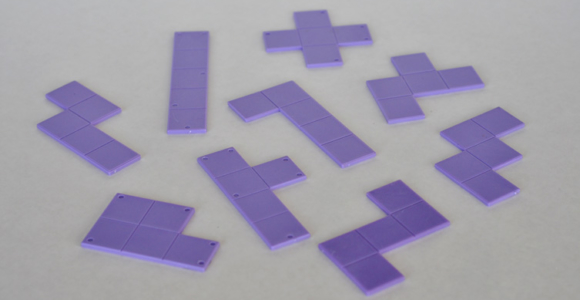
Pentominoes
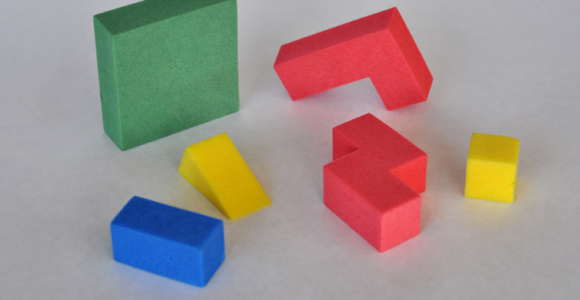
Dime Solids